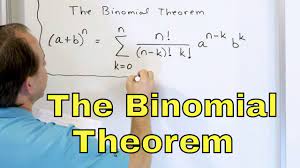
The Binomial Theorem, also known as binomial expansion, describes the algebraic expansion of binomial powers. To understand it better, we’ll first define a binomial and see how the binomial theorem applies to everyday activities.
A monomial is the most basic type of polynomial in mathematics. A binomial is generally written as axm + bxn, where axm and bxn are monomials that add up to form the binomials. Here, a and b are numbers, m and n are non-negative distinct integers, and x is a variable with an unfixed value. What exactly is the binomial theorem? As the power increases, the expansion becomes more difficult to calculate. The Binomial Theorem can be used to easily calculate a binomial expression that has been raised to a very large power.
The Binomial Expansion’s Terms
Typically, the binomial expansion is asked to find the middle term or the general term. There are several terms in the binomial expansion that are covered here:
- General Terms
- Middle Terms
- Separate Term
- Choosing a Specific Term
- The greatest term in terms of numbers
- Consecutive Terms/Coefficients Ratio
What is a Binomial Expression?
A binomial expression is defined as a mathematical expression that consists of two terms. Furthermore, these two terms must be separated by either addition or subtraction. To add the binomials, combine equal terms to obtain an answer. The distributive property should be used to multiply the binomials.
Formula for the Binomial Theorem
The Binomial Theorem is a quick way to expand or multiply a binomial expression. The expression has also been elevated to a higher level of significance. As we all know, multiplying such expressions with large powers is always difficult. However, the Binomial expansions and their formulas are extremely useful in this regard. The Binomial theorem and its Formula will be discussed in this article.
| (a+b)n=k=0n(kn)akbn-k |
Properties Of Binomial Theorem
- There are n+1 terms in total.
- (x)n is the first known term.
- The exponent for x decreases by one from term to term as we progress from the first to the last term. In contrast, the exponent of y increases by one term. In addition, the sum of the exponents in each term will be n.
- We can easily obtain the coefficient for the next term by multiplying the coefficient of each term by the exponent of x in that term and dividing the product by the number of that term.
Binomial Theorem In Daily Life
- The binomial theorem is frequently used in statistical and probability analyses.
- It is extremely useful because our economy is based on statistical and probability analyses.
- The Binomial Theorem is used in higher mathematics and calculation to find roots of equations in higher powers.
- It is also used in the proof of many important equations in physics and mathematics.
Importance of Binomial Theorem in Higher Mathematics
In calculation as well as higher mathematics, the binomial theorem is used to solve highly complex and nearly impossible calculations. Many of Sir Albert Einstein’s equations, laws, and theories made extensive use of binomial theorems. If you want to use the Binomial Theorem in advanced scenarios, your current knowledge of mathematics may not be sufficient. However, if you are already interested in learning about higher mathematics, the concepts of this theorem will be extremely useful for conducting any type of research or mathematical analysis and projects.
Math Online Classes
Math is a fascinating subject. Math, on the other hand, has never been an easy subject. Cuemath can help you lay a solid foundation in this subject. If you do, Cuemath can assist you. It offers extremely low-cost online math classes. The best thing about math online classes is that they force you to visualise different problems, which aids in conceptual clarity.